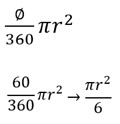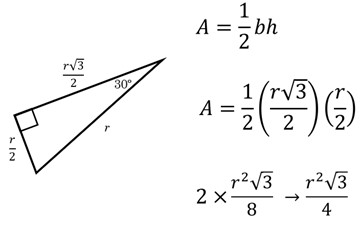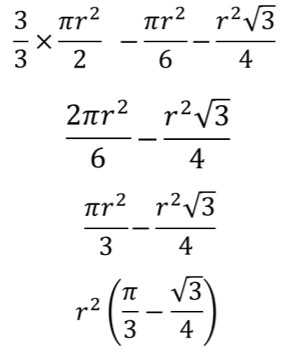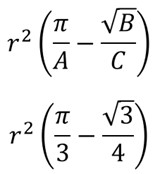This is a super cool problem, I love it! Here’s how we will tackle this one.
- Go over the details and demands of the problem.
- Offer three clues (which you can skip).
- Run through the solution.
- Celebrate!
The Set-Up
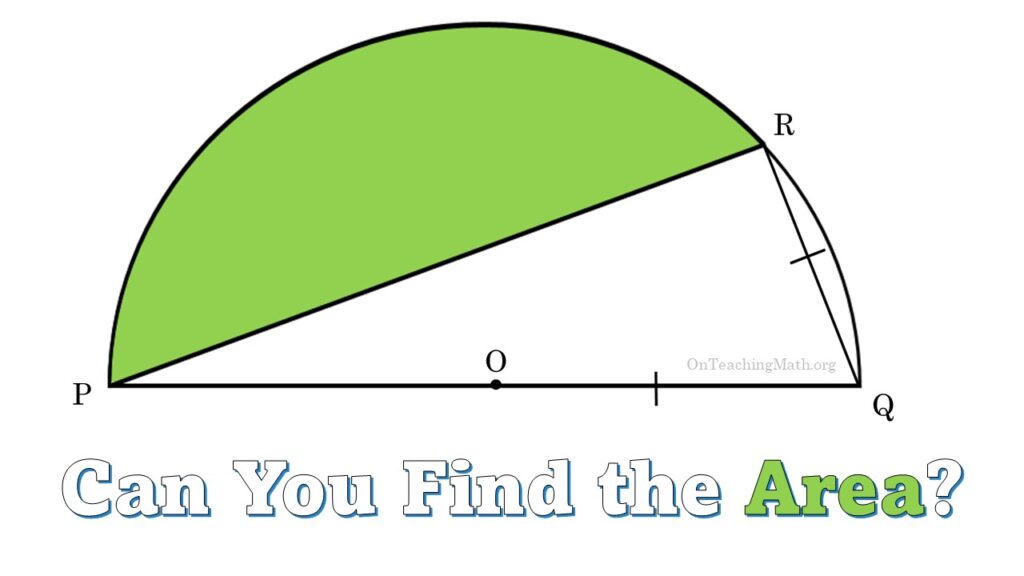
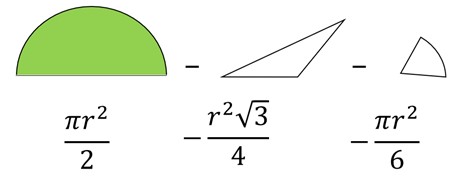
The diagram is not drawn to scale, but here’s what’s at play. There’s a semicircle with center O, and radius OQ. RQ is congruent to OQ. There’s a triangle inscribed in the semicircle with points PRQ. The region above the triangle is shaded in green and we need to find its area.
Clue #1
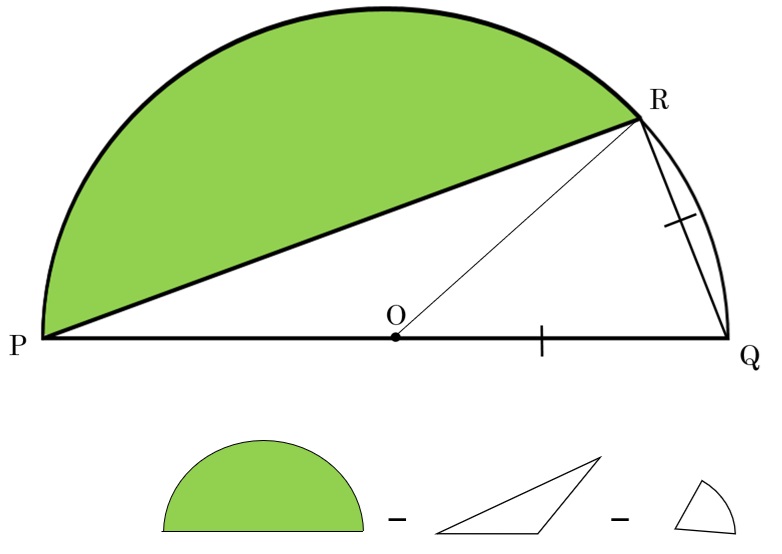
Can you write a formula, or draw a diagram, of the composite shape that separates the green region from the semicircle? If you started with the semicircle and then subtracted a sector and triangle POR (now shown in the original diagram), what would remain is the green region.
Clue #2
The sector ROQ has a central angle that is 60 degrees.
Clue #3
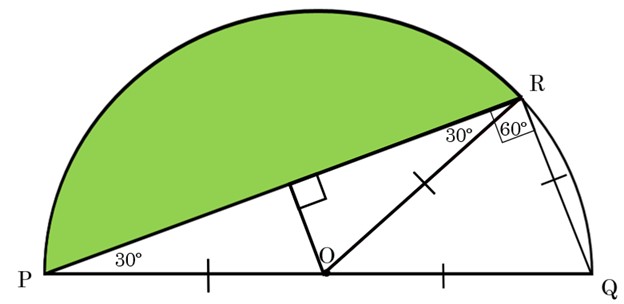
Triangle PRQ is a right triangle because it lies on the diameter and is inscribed. Triangle ROQ is equilateral. Armed with this information you can find the angles of triangle POR.
Solution
Let’s start by showing that the green region is the semicircle minus both triangle POR and sector ROQ, as shown in the diagram below.
We will find the area of each portion, and then combine them to get at our solution.
Let’s start with sector ROQ.
Since OR is the radius, and RQ is congruent to OQ, triangle ROQ is equilateral. So, the angle ROQ is 60-degrees. We can find the area of the sector as shown below.
Now let’s tackle triangle POR.
Since ROQ is a 60-degree angle and PRQ is a 90-degree angle, angle PR0 is a 30-degree angle! That makes triangle POR an isosceles triangle! We can drop a perpendicular bisector giving us a nice 30-60-90 right triangle with the hypotenuse being the radius of the semicircle. How sweet is that? Really, I mean, come on! If only all of life’s problems were set up to be solved with such beautiful arrangements and discovery. This feels like a real-life Magnum PI episode!
Using the properties of the 30-60-90 we can find the sides, and then the area of the triangle shown below. Since there are two of these little guys that make up triangle POR, we multiple the result by two.
Now we have the two difficult pieces in place. The semicircle is of course half of the circle, so “pi-r-squared divided by two.”
Here’s what we have.
Our answer needs to be in the form shown below, so we will rearrange our information so that the terms with pi are together and the term with the square root are at the end of the expression.
The subsequent steps are often daunting to students, but involve common denominators and reducing, that’s it. A little courage combined with care are all that are required.
By writing our answer in the form required by the problem we can see that A is 3, as is B, while C is 4.
I really hope you enjoyed this problem. I did!
If you’re a math teacher, I’d like to encourage you to use this in your classroom. I post a weekly math for fun problem. Students are not incentivized to work on them, just invited passively. Those that decide to tackle the problem do so for the fun of it, nothing more. I think it’s a great way to steal some fun and enjoyment of learning back from the tyrannical pressure of grades, GPA, and scholarship opportunities.
If you’d like to use this problem in your room, use the links below.
Until next time, this is Philip Brown, signing off!
